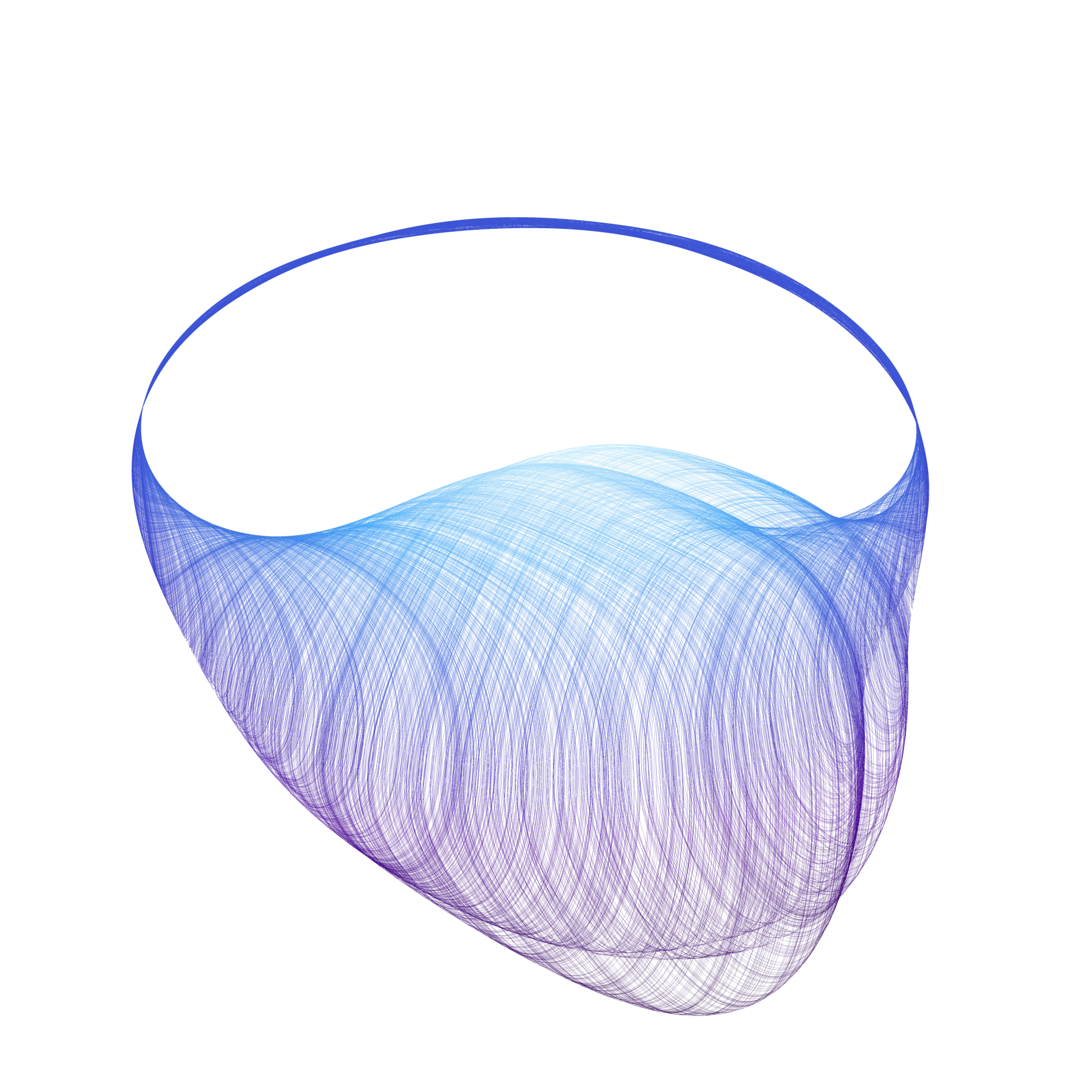
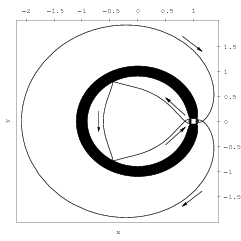
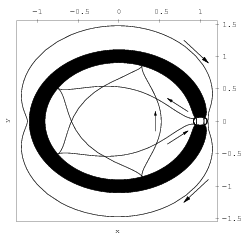
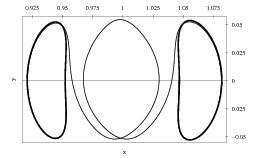
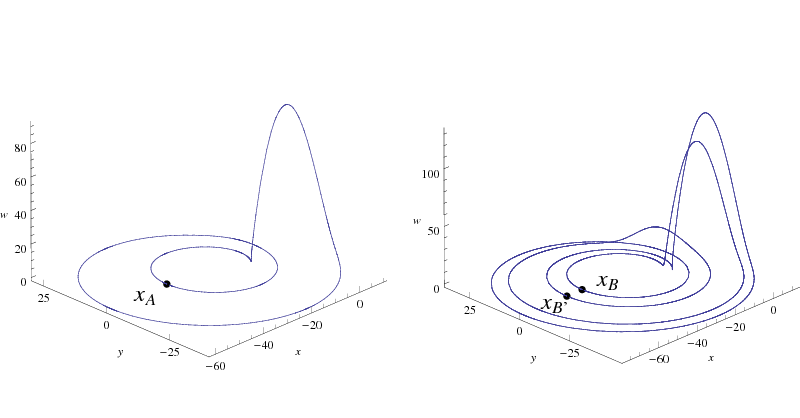
Homoclinic and heteroclinic connections of hyperbolic objects play an important role in the study of dynamical systems from a global point of view. They were used in the design of space missions using libration point dynamics, among which the Genesis has been the first one to make use of a heteroclinic connection. For the design of such missions, the circular Restricted Three Body Problem (RTBP) is the natural problem to start with. Of special interest are the L₁ and L₂ libration points because of their suitability to place stationary satellites.

In [1] we proposed a method for rigorous verification of the existence of homoclinic and heteroclinic points for planar maps. The method has been applied to the Henon map [1] and to the Planar Restricted Three Body Problem [2,3]. Using validated solvers from the CAPD for ODE's and first order variational equations we were able to prove the existence of symbolic dynamics, homoclinic and heteroclinic solutions between Lyapunov periodic orbits for parameter values corresponding to the Oterma comet in the Sun-Jupiter system.

Later [4,5] the method has been generalized to any finite dimensions and successfully applied to the hyperchaotic Rossler system [6] were the proof of the existence of infinitely many of homoclinic and heteroclinic solutions between periodic orbits is given.

1. Z. Galias, P. Zgliczyński, Abundance of homoclinic and heteroclinic orbits and rigorous bounds for the topological entropy for the Henon map, Nonlinearity, 14, 909-932 (2001).
2. D. Wilczak, P. Zgliczyński, Heteroclinic Connections between Periodic Orbits in Planar Circular Restricted Three Body Problem - A Computer Assisted Proof, Communications in Mathematical Physics, Vol.234, No.1, 37-75 (2003).
3. D. Wilczak, P. Zgliczyński, Heteroclinic Connections between Periodic Orbits in Planar Circular Restricted Three Body Problem - part II, Communications in Mathematical Physics, Vol. 259, No.3, 561-576 (2005).
4. H. Kokubu, D. Wilczak, P. Zgliczyński, Rigorous verification of cocoon bifurcations in the Michelson system, Nonlinearity 20, No.9, 2147-2174 (2007).
5. P. Zgliczyński, Covering relations, cone conditions and the stable manifold theorem, J. of Diff. Equations 246 (2009) 1774-1819.
6. D. Wilczak, Abundance of heteroclinic and homoclinic orbits for the hyperchaotic Rossler system, Discrete and Continuous Dynamical Systems - Series B, Vol. 11, No. 4, 1039-1055 (2009).

Two pairs of verified homoclinic orbits to Lyapunov orbits L₁ and L₂.

Heteroclinic solution connecting these periodic orbits. The connection in the opposite direction follows from the reversibility of the system.

Two periodic solutions for the 4D Rossler system of periods 2 and 4 in the Poincare map. We proved the existence of infinitely many homoclinic and heteroclinic solutions between them.